“Truth can be stated in a thousand different ways, yet each one can be true…”
Swami Vivekananda
What is ‘Test of Hypothesis’?
Ø Test of Hypothesis (Hypothesis Testing) is a process of testing of the significance regarding the parameters of the population on the basis of sample drawn from it.
Ø Test of hypothesis is also called as ‘Test of Significance’.
Ø J. Neyman and E.S. Pearson initiated the practice of testing of hypothesis in statistics.
What is the purpose of Hypothesis Testing?
Ø The main purpose of hypothesis testing is to help the researcher in reaching a conclusion regarding the population by examining a sample taken from that population.
Ø The hypothesis testing does not provide proof for the hypothesis.
Ø The test only indicates whether the hypothesis is supported or not supported by the available data.
What is Hypothesis?
Ø Hypothesis is a statement about one or more populations.
Ø It is a statement about the parameters of the population about which the statement is made.
Ø Example:
$ A doctor hypothesized: “The drug ‘X’ is ineffective in 99% of cases of which it is used”.
$ “The average pass percentage of central university degree programme is 98”.
Ø Through the hypothesis testing the researcher or investigator can determine whether or not such statements are compatible with the available data.
| You may also like NOTES in... | ||
|---|---|---|
| BOTANY | BIOCHEMISTRY | MOL. BIOLOGY |
| ZOOLOGY | MICROBIOLOGY | BIOSTATISTICS |
| ECOLOGY | IMMUNOLOGY | BIOTECHNOLOGY |
| GENETICS | EMBRYOLOGY | PHYSIOLOGY |
| EVOLUTION | BIOPHYSICS | BIOINFORMATICS |
Types of Hypothesis
Ø There are TWO types of hypothesis.
(A). Research Hypothesis
(B). Statistical Hypothesis
(A). Research Hypothesis
Ø Research Hypothesis is “a tentative solution for the problem being investigated”.
Ø It is the supposition (guess) that motivates the research.
Ø In research, the researcher determines whether or not their supposition can be supported through scientific investigation.
Ø The research hypothesis directly leads to statistical hypothesis.
(B). Statistical Hypothesis
Details of the Statistical hypothesis are discussed in the “Steps or Components in Testing of Statistical Hypothesis”.
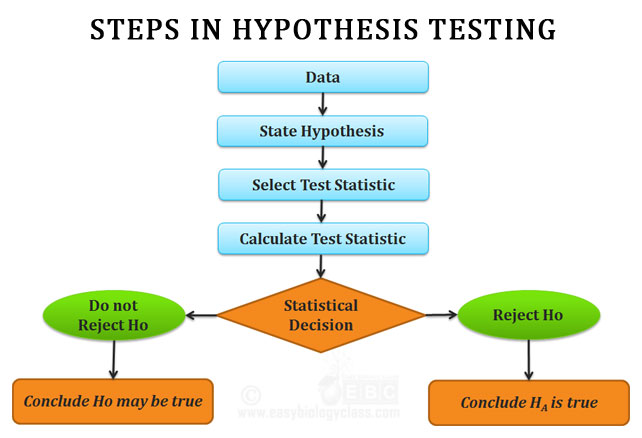
Steps / Components in Testing of Statistical Hypothesis:
Ø The statistical hypothesis testing consists of following Steps / Components
(1). Data (variable)
(2). Statistical Hypothesis
(3). Test Statistic
(4). Decision Rule
(5). Significance Level
(6). Statistical Decision
(7). p – Value
(1). Data (variable)
Ø Data is the information collected from the population.
Ø It may be the observation of a natural phenomenon, Result of an experiment, Data from a survey or a secondary data.
Ø The nature of data determines the type of statistical test to be selected.
Ø All the features of the data such as continuous, discontinuous, quantitative or qualitative etc. matters in the process of hypothesis testing.
(2). Statistical Hypothesis
Ø Statistical hypothesis is a statement about the population which we want to verify on the basis of information available from the sample.
Ø A statistical hypothesis is stated in such a way that they may be evaluated by appropriate statistical techniques.
Ø There are TWO types of statistical hypothesizes:
(a). Null hypothesis
(b). Alternative hypothesis
(a). Null Hypothesis
Ø The Null hypothesis is the hypothesis to be tested by test statistic.
Ø Null hypothesis is denoted as H0.
Ø Usually the null hypothesis stated as the ‘Hypothesis of No Difference’.
Ø The statement is created complementary to the conclusion that the researcher is seeking to reach through his research.
Ø Usually stated in the negative terms of the original research hypothesis.
Ø Example: The drug ‘X’ DO NOT induces apoptosis in cancerous cells.
Ø In the statistical testing process, the null hypothesis is either:
$ Rejected
$ Not rejected (Fail to be rejected / accepted)
Ø If the null hypothesis is not rejected, we say that the data on which the test is based do not provide sufficient evidence to cause the rejection of null hypothesis.
Ø If the null hypothesis is rejected in the testing process, we say that the data at hand are not compatible with the null hypothesis but are supportive for some other hypothesis (commonly called as alternative hypothesis).
(b). Alternative Hypothesis
Ø Alternate hypothesis is created in a negative meaning of the null hypothesis.
Ø It is denoted as H1 or HA.
Ø Usually the alternative hypothesis and research hypothesis are the same.
Ø Example: The drug ‘X’ induces apoptosis in cancerous cells.
How to state the statistical hypothesis?
Ø The null hypothesis should contain an equality sign (=, ≤ or ≥).
Ø Example: The population mean (μ) is not 100.
$ H0: μ = 100
$ H1: μ ≠ 100
Ø Example: The population means is greater than 100.
$ H0: μ ≤ 100
$ H1: μ > 100
Ø Example: The population mean is less than 100.
$ H0: μ ≥ 100
$ H1: μ < 100
Things to remember when constructing the Null Hypothesis:
$ What you expected to conclude with the study should be placed in the alternative hypothesis.
$ The null hypothesis should contain a statement of equality (=, ≤, ≥).
$ The null hypothesis is the hypothesis to be tested.
$ The null hypothesis and alternative hypothesis should be complementary.
(3). Test Statistic
Ø Test statistic is the statistic computed from the data sample.
Ø There are many possible values that the test statistic can adopt.
Ø Test value of the statistic depends on the nature of the sample.
Ø The test statistic is the decision maker in hypothesis testing.
Ø Decision is to reject or not reject the null hypothesis.
Ø General formula for test statistic: (applicable to most of the test statistic but not to all)
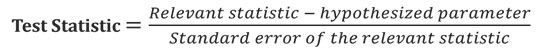
Example:

Where:
x̄ : mean
μ0 : hypothesized value of population mean
σ/√n : Standard error
(4). Decision Rule
Ø All the possible values that the test statistic can assume are points on the horizontal axis of a graph of the distribution of the test statistic.
Ø The values are divided into two groups:
1. Values of the rejection region
2. Values of the non-rejection region
Ø The decision rule tell us to reject the null hypothesis if the values of the test statistic that we compute from our sample is one of the values in the rejection region and not to reject the null hypothesis if the computed values of the test statistic is on the values in the non-rejection region.
(5) Significance Level
Ø Level of significance is the probability of rejecting a true null hypothesis in the statistical testing procedure.
Ø The level of significance is a probability value and it is denoted as ‘α’.
Ø The significance level decide the decision value to go the rejection region or to the non-rejection region.
Ø Due to the ‘Level of significance’ the test statistic is often called as ‘Significance Test’.
Ø If we reject a true null hypothesis we are committed an error.
Ø Thus, you have to ensure that the probability of rejecting a true null hypothesis is very small.
Ø Thus, we select a small value of α to ensure the probability of rejecting a true null hypothesis is very less.
Ø The frequently used α values are 0.01 (99%), 0.05 (95%).
Ø Explanation: if we select 0.01 (99%) as the significance level, it means that we are 99% confident in our decision but still there is 1% change for our decision being false.
(6). Statistical Decision
Ø It is the decision of rejecting or not rejecting the null hypothesis.
Ø We reject the null hypothesis if the computed value of the test statistic is fall in the rejection region.
Ø We will NOT reject the null hypothesis if the computed value falls in the non-rejection region.
Ø Conclusion:
Ø If we reject H0, we conclude that HA is true.
Ø If we fail to reject H0, we conclude that the H0 may be true.
Ø When a null hypothesis is not rejected one should not say that the null hypothesis accepted but we say that null hypothesis is not rejected.
Ø We usually avoid the usage ‘accept’, because we may have committed a type II error.
Learn more: Statistical Errors (Type I and Type II Errors)
(7). p-Value
Ø p-value is the smallest value of α for which we can reject a null hypothesis.
Ø A p-value is the probability that the computed value for a test statistic is at least as extreme as specified value of the test statistic when the null hypothesis is true.
| You may also like... | ||
|---|---|---|
| NOTES | QUESTION BANK | COMPETITIVE EXAMS. |
| PPTs | UNIVERSITY EXAMS | DIFFERENCE BETWEEN.. |
| MCQs | PLUS ONE BIOLOGY | NEWS & JOBS |
| MOCK TESTS | PLUS TWO BIOLOGY | PRACTICAL |
Reference
Daniel, W.W., 1999. Biostatistics: A foundation for analysis in the health sciences 9th edition. John Wiley & Sons inc.: USA.
Khan, I.A. and Khanum, A., 2012, Fundamentals of Biostatistics, 3rd edition (revised), Ukaaz Publications, Hyderabad, India.
Kothari, C.R., 2004. Research methodology: Methods and techniques. New Age International, India.
<<< Back to Statistics Note Papte
Do you have any Queries?
Please leave me a COMMENT in the Comments Section below.
I will be Happy to Read your Comments and Reply.
Download the PPT of this Topic
You might also like…
@. Introduction to Biostatistics
@. Data Collection Methods in Statistics
@. Difference between Type I and Type II Errors
More Lecture Notes from Easy Biology Class…
BotanyZoologyBiochemistryGeneticsMolecular BiologyBiotechnologyEndocrinologyPhysiologyMicrobiologyImmunologyEmbryologyEcologyEvolutionBiophysicsResearch MethodologyBiostatisticsChemistry for BiologistsPhysics for Biologists
Browse more in Easy Biology Class…
Lecture NotesBiology PPTsVideo TutorialsBiology MCQQuestion BankDifference betweenPractical AidsMock Tests (Online)Biology Exams